Secrets of Mental Math, Arthur Benjamin [management books to read .txt] 📗

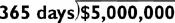
First you must determine the magnitude of the answer. Does this player earn thousands every day? Well, 365 × 1000 = 365,000, which is too low.

Does he earn tens of thousands every day? Well, 365 × 10,000 = 3,650,000, and that’s more like it. To guesstimate your answer, divide the first two digits (or 36 into 50) and figure that’s 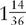or 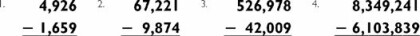Since 18 goes into 70 about 4 times, your guess is that the athlete earns about $14,000. The exact answer is $13,698.63 per day. Not a bad estimate (and not a bad salary!).

Here’s an astronomical calculation for you. How many seconds does it take light to get from the sun to the earth? Well, light travels at 186,282 miles per second, and the sun is (on average) 92,960,130 miles away. I doubt you’re particularly eager to attempt this problem by hand. Fortunately, it’s relatively simple to guesstimate an answer. First, simplify the problem:

Now divide 186 into 930, which yields 5 with no remainder. Then append the two 0s you removed from 93,000 and you get 500 seconds. The exact answer is 499.02 seconds, so this is a very respectable guesstimate.

MULTIPLICATION GUESSTIMATION

You can use much the same techniques to guesstimate your answers to multiplication problems. For example,

Rounding up to the nearest multiple of 10 simplifies the problem considerably, but you’re still off by 252, or about 5%. You can do better if you round both numbers by the same amount, but in opposite directions. That is, if you round 88 by increasing 2, you should also decrease 54 by 2:

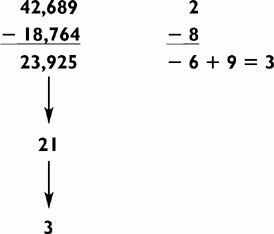
Instead of a 1-by-1 multiplication problem, you now have a 2-by-1 problem, which should be easy enough for you to do. Your guesstimation is off by only 1.5%.

When you guesstimate the answer to multiplication problems by rounding the larger number up and the smaller number down, your guesstimate will be a little low. If you round the larger number down and the smaller number up so that the numbers are closer together, your guesstimate will be a little high. The larger the amount by which you round up or down, the greater your guesstimate will be off from the exact answer. For example:

Since the numbers are closer together after you round them off, your guesstimate is a little high.

Since the numbers are farther apart, the estimated answer is too low, though again, not by much. You can see that this multiplication guesstimation method works quite well. Also notice that this problem is just 672 and that our approximation is just the first step of the squaring techniques. Let’s look at one more example:

We observe that the approximation is most accurate when the original numbers are close together. Try estimating a 3-by-2 multiplication problem:

By rounding 63 down to 60 and 728 up to 731, you create a 3-by-1 multiplication problem, which puts your guesstimate within 2004 of the exact answer, an error of 4.3%.

Now try guesstimating the following 3-by-3 problem:

You will notice that although you rounded both numbers up and down by 8, your guesstimate is off by over 1000. That’s because the multiplication problem is larger and the size of the rounding number is larger, so the resulting estimate will be off by a greater amount. But the relative error is still under 1%.

How high can you go with this system of guesstimating multiplication problems? As high as you want. You just need to know the names of large numbers. A thousand thousand is a million, and a thousand million is a billion. Knowing these names and numbers, try this one on for size:

As before, the objective is to round the numbers to simpler numbers such as 29,000,000 and 14,000. Dropping the 0s for now, this is just a 2-by-2 multiplication problem: 29 × 14 = 406 (29 × 14 = 29 × 7 × 2 = 203 × 2 = 406). Hence the answer is roughly 406 billion, since a thousand million is a billion.

SQUARE ROOT ESTIMATION: DIVIDE AND AVERAGE

The quantity , the square root of a number n, is the number which, when multiplied by itself, will give you n For example, the square root of 9 is 3 because 3 × 3 = 9. The square root is used in many science and engineering problems and is almost always solved with a calculator. The following method provides an accurate estimate of the answer.

In square root estimation your goal is to come up with a number that when multiplied by itself approximates the original number. Since the square root of most numbers is not a whole number, your estimate is likely to contain a fraction or decimal point.

Let’s start by guesstimating the square root of 19. Your first step is to think of the number that when multiplied by itself comes closest to 19. Well, 4 × 4=16 and 5 × 5 = 25. Since 25 is too high, the answer must be 4 point something. Your next step is to divide 4 into 19, giving you 4.75. Now, since 4 × 4 is less than 4 × 4.75 = 19, which in turn is less than 4.75 × 4.75, we know that 19 (or 4 × 4.75) lies between 42 and 4.752. Hence, the square root of 19 lies between 4 and 4.75.

I’d guess the square root of 19 to be about halfway between, at 4.375. In fact, the square root of 19 (rounded to three decimal places) is 4.359, so our guesstimate is pretty close. We illustrate this procedure as follows:

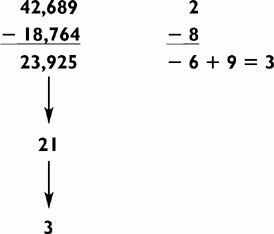
Actually, we can obtain this answer another way, which you might find easier. We know 4 squared is 16, which is shy of 19 by 3. To improve our guess, we “add the error divided by twice our guess.” Here, we add 3 divided by 8 to get 4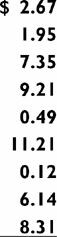 = 4.375. We note that this method will always produce an answer that is a little higher than the exact answer.

Now you try a slightly harder one. What’s the square root of 87?

First come up with your ballpark figure, which you can get fairly quickly by noting that 9 × 9 = 81 and 10 × 10 = 100, which means the answer is 9 point something. Carrying out the division of 9 into 87 to two decimal places, you get 9.66. To improve your guesstimate, take the average of 9 and 9.66, which is 9.33—exactly the square root of 87 rounded to the second decimal place! Alternatively, our guesstimate is 9 + (error)/18 = .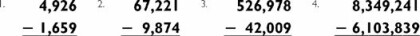

Using this technique, it’s pretty easy to guesstimate the square root of two-digit numbers. But what about three-digit numbers? Actually, they are not much harder. I can tell you right off the bat that all three-digit and four-digit numbers have two-digit square roots before the decimal point. And the procedure for computing square roots is the same, no matter how large the number. For instance, to compute the square root of 679, first find your ballpark figure. Because 20 squared is 400 and 30 squared is 900, the square root of 679 must lie between 20 and 30.

When you divide 20 into 679, you get approximately 34. Averaging 20 and 34 gives you a guesstimate of 27, but here’s a better estimate. If you know that 25 squared is 625, then your error is 679 − 625 = 54. Dividing that by 50, we have = 1.08. Hence our improved guesstimate is 25 + 1.08 = 26.08. (For an even better estimate, if you know that 26 squared is 676, your error is 3, so add .06 to get 26.06.) The exact answer is 26.06, rounded to two decimal places.

To guesstimate the square root of four-digit numbers, look at the first two digits of the number to determine the first digit of the square root. For example, to find the square root of 7369, consider the square root of 73. Since 8 × 8 = 64 and 9 × 9 = 81, 8 must be the first digit of the square root. So the answer is 80 something. Now proceed the usual way. Dividing 80 into 7369 gives 92 plus a fraction, so a good guesstimate is 86. If you squared 86 to get 7396, you would be high by 27, so you should subtract .16 for a better guesstimate of 85.84, which is right on the money.

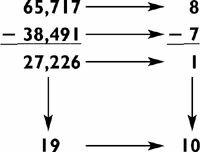
To guesstimate the square root of a six-digit number like 593,472 would seem like an impossible task for the uninitiated, but for you it’s no sweat. Since 7002 = 490,000, and 8002 = 640,000, the square of 593,472 must lie between 700 and 800. In fact, all five-digit and six-digit numbers have three-digit square roots. In practice, you only need to look at the square root of the first two digits of six-digit numbers (or the first digit of five numbers). Once you figure out that the square root of 59 lies between 7 and 8, you know your answer is in the 700s.

Now proceed in the usual manner:

The exact square root of 593,472 is 770.37 (to five places), so you’re pretty close. But you could have been closer, as the following trick demonstrates. Note that the first two digits, 59, are closer to 64 (8 × 8) than they are to 49 (7 × 7). Because of this you can start your guesstimation with the number 8 and proceed from there:

Just for fun, let’s do a real whopper—the square root of 28,674,529. This isn’t as hard as it might seem. Your first step is to round to the nearest large number—in this case, just find the square root of 29.

All seven-digit and eight-digit numbers have four-digit square roots, so 5.4 becomes 5400, your estimate. The exact answer is slightly greater than 5354.8. Not bad!

This wraps up the chapter on guesstimation math. After doing the exercises below, turn to the next chapter on pencil-and-paper math, where you will learn to write down answers to problems, but in a much quicker way than you’ve done on paper before.

The Mathematical Duel of Évariste Galois

The tragic story of the French mathematician Évariste Galois (1811–1832) killed at the age of twenty in a duel over “an infamous coquette” is legendary in the annals of the history of mathematics. A precociously brilliant student, Galois laid the foundation for a branch of mathematics known as group theory. Legend has it that he penned his theory the night before the duel, anticipating his demise and wanting to leave his legacy to the mathematics community. Hours before his death, on May 30, 1832, Galois wrote to Auguste Chevalier: “I have made some new discoveries in analysis. The first concerns the theory of equations, the others integral functions.” After describing these, he asked his friend: “Make a public request of Jacobi or Gauss to give their opinions not as to the truth but as to the importance of these theorems. After that, I hope some men will find it profitable to sort out this mess.”

Romantic legend and historical truth, however, do not always match. What Galois penned the night before his death were corrections and editorial changes to papers that had been accepted by the Academy of Sciences long before. Further, Galois’s initial papers had been submitted three years prior to the duel, when he was all of seventeen! It was after this that Galois became embroiled in political controversy, was arrested, spent time in a prison dungeon, and, ultimately, got himself mixed up in a dispute over a woman and killed.

Aware of his own precocity, Galois noted, “I have carried out researches which will halt many savants in theirs.” For over a century that proved to be the case.

MORE TIPS ON TIPS

As we indicated in Chapter 0, it is easy to figure out tips for most situations. For example, to figure out a 10% tip, we merely multiply the bill by 0.1 (or divide